Nfp 104 15 20 2014-2025 Form


What is the Form Nfp 104 15 20
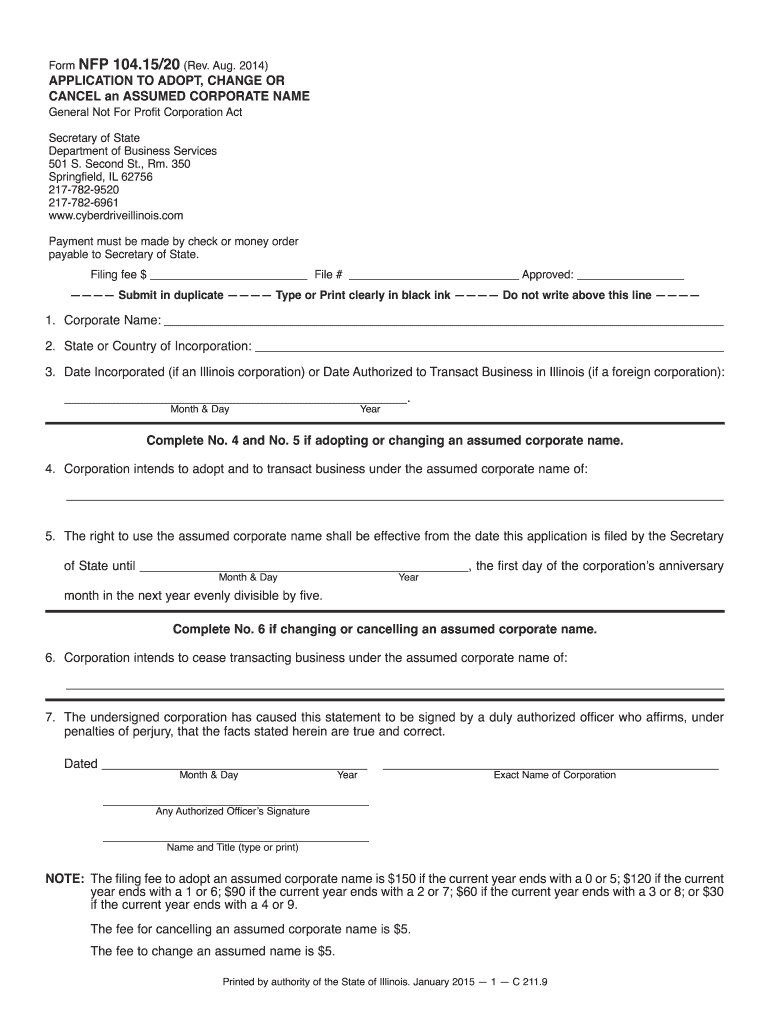
The Nfp 104 15 20 is a form used in Illinois for the adoption and cancellation of assumed corporate names. This form is essential for businesses that wish to register a name that differs from their legal business name. It allows organizations to operate under a different name while maintaining legal compliance. The form is specifically designed for non-profit organizations but can also be applicable to other business entities seeking to adopt or cancel an assumed name.
How to use the Form Nfp 104 15 20
To use the Nfp 104 15 20, businesses must fill out the required sections accurately. This includes providing the legal name of the organization, the assumed name to be adopted or canceled, and the address of the principal office. It is important to ensure that the assumed name complies with state regulations and does not infringe on existing trademarks. Once completed, the form must be submitted to the appropriate state office for processing.
Steps to complete the Form Nfp 104 15 20
Completing the Nfp 104 15 20 involves several key steps:
- Download the form from the official state website or obtain a physical copy.
- Fill in the organization’s legal name and address.
- Specify the assumed name you wish to adopt or the name you wish to cancel.
- Provide any additional required information, such as the date of adoption or cancellation.
- Sign and date the form, ensuring that all information is accurate.
- Submit the form to the Illinois Secretary of State's office either online or by mail.
Legal use of the Form Nfp 104 15 20
The Nfp 104 15 20 must be used in accordance with Illinois state law. This includes ensuring that the assumed name is not misleading and complies with the naming conventions established by the state. Legal use of the form also requires that the organization has the authority to adopt or cancel the name, which is typically outlined in the organization's bylaws or articles of incorporation.
Required Documents
When submitting the Nfp 104 15 20, certain documents may be required to support the application. These can include:
- A copy of the organization's articles of incorporation or bylaws.
- Proof of the assumed name's availability, which can be verified through a name search.
- Any previous filings related to the assumed name, if applicable.
Form Submission Methods
The Nfp 104 15 20 can be submitted through various methods, including:
- Online submission via the Illinois Secretary of State's website.
- Mailing a physical copy of the completed form to the appropriate office.
- In-person submission at designated state offices.
Quick guide on how to complete nfp 104 15 20 change name
Administer nfp 104 15 20 change name anytime, anywhere
Your regular business activities might require extra attention when handling state-specific business documents. Regain your office time and cut down on paper costs associated with document-driven processes using airSlate SignNow. airSlate SignNow provides a wide array of pre-loaded business documents, including nfp 104 15 20, which you can utilize and distribute with your business associates. Handle your cancel assumed corporate seamlessly with powerful editing and eSignature features and send it directly to your recipients.
Steps to obtain adopt cancel assumed in just a few clicks:
- Select a form applicable to your state.
- Click Discover More to access the document and verify its accuracy.
- Press Acquire Form to begin using it.
- application adopt assumed will automatically open in the editor. No further actions are required.
- Utilize airSlate SignNow’s sophisticated editing tools to complete or adjust the form.
- Click on the Sign option to create your personal signature and eSign your document.
- When prepared, click Finish, save your changes, and access your document.
- Disseminate the form via email or text, or use a link-to-fill method with your associates or allow them to download the document.
airSlate SignNow greatly reduces the time spent managing [SKS] and allows you to locate necessary documents in one place. An extensive library of forms is organized and designed to support essential business operations needed for your enterprise. The advanced editor decreases the likelihood of errors, enabling you to easily correct mistakes and review your documents on any device prior to sending them out. Start your complimentary trial today to explore all the advantages of airSlate SignNow for your daily business workflows.
Create this form in 5 minutes or less
FAQs nfp 104 15 20 cancel
-
If I was at a Casino and lost over $20,000 in a slot machine before hitting a $10,000 jackpot, will I still have to fill out a tax form and declare that as income even thought I really lost $10,000?
There are two ways to handle slot winnings/losses. The “regular” way is that all winnings (whether you receive a W-2G or not) are reported on your 1040 as “other income”, and your losses that you can substantiate are deducted on your Schedule A (assuming that you itemize, etc.). Under that method, yes, you would report the $10K jackpot (plus any other winnings that came out of the machine(s)), and you would deduct your losses up to the amount of your reported winnings as an itemized deduction.The other way to do it is the technically correct way — and that is to net your slot results on a “session” basis. That is, from the time that you pull your first lever (or push your first “spin” button”) of the day until you stop playing the slots, other than short breaks — but not beyond midnight — you total your net winnings/losses, and that’s your winnings or losses for the “session” that are reportable on your tax return. That method, for example, enables you to take advantage of losses even if you don’t itemize deductions. Now, that’s not going to match up with any W-2G’s that you get, because the casinos don’t report on that basis (they don’t even report on the basis of a midnight-to-midnight day). But the IRS has ways in which you can indicate that on your return.So the answer to your question is, maybe. It depends on (i) when did you lose the $20K and win the $10K?, (ii) what kind of records do you have (a “players’ card” statement would be great), and (iii) what else did you win gambling?
-
In Bingo, where you have 15 out of 90 numbers, a grid 3 by 9, you must have a least 1 number from every group, 1 to 9, 10 to 19, 20 to 29 etc, and no more than 3 numbers from each. What is the math to work out how many permutations there are ?
Please explain the role of the 3x8 grid. Normal bingo cards/grids are 5x5. If you are thinking that each column contains a unique group (using your terminology), then you need a 3x9 group.EDIT: I thought a bit about your question this morning and think I have a guess at what you were trying to ask. Let me see if this is correct.You have a total of 90 balls each with a unique number. You also have a grid 9 rows and 3 columns. You draw 15 balls at random and place them on the grid based on which group (1–9, 10–10, 20–29, … , 80–90). You want to know how may ways the grid can be filled in where there is at least 1 ball in each row but no more than 3. Because you used the word permutation, I’m going to assume that you do care about the order of the balls in each row, i.e. 20,26,23 is not the same as 23,26,20.Before answering this, I’m going to suggest that we redefine the groups as 1–10, 2–20, …, 81–90. Otherwise, there is a nasty asymmetry occurring at the endpoints. The first row only has 9 possible balls to fill it. The last row has 11. All other rows have 10. This tweak will make the math a whole lot easier.So, on to the solution…The first step is to figure out how many ways you can partition the 15 balls into 9 groups of 1, 2, or 3 balls. We know that each of the 9 groups must contain at least 1 ball. This means we must distribute the remaining 6 balls into groups of 1 or 2 balls each. There are 4 possible ways to do this: {2,2,2}, {2,2,1,1},{2,1,1,1,1},{1,1,1,1,1,1}. These correspond to 4 partitions of the 15 balls into 9 groups of 1, 2, or 3 balls: {3,3,3,1,1,1,1,1,1}, {3,3,2,2,1,1,1,1,1},{3,2,2,2,2,1,1,1,1},{2,2,2,2,2,2,1,1,1}.The second step is to figure out how to distribute each of these partitions to the actual rows in the grid. But, we must address each on its own.The first partition contains three 3’s and six 1’s. There are C(9,3) = (9*8*7)/(3*2*1) = 84 ways to pick the three rows which will contain the 3’s. There is only 1 way to pick the six rows to contain the 1’s. This gives a total of 84 patterns associated with this partition.The second partition contains two 3’s, two 2’s and five 1’s. There are C(9,2) = (9*8)/(2*1) = 36 ways to pick the 2 rows which will contain the 3’s. There are C(7,2) = (7*6)/(2*1) = 21 ways to pick the 2 rows will will contain the 2’s. Again, there is only 1 way to pick the 5 rows to contain the 1’s. This gives a total of 36*21 = 756 patterns associated with this partition.The third partition contains one 3, four 2’s and four 1’s. There are C(9,1) = 9 ways to pick the row which will contain the 3. There are C(8,4)=(8*7*6*5)/(4*3*2*1) = 70 ways to pick the four rows will will contain the 2’s. Again, there is only one way to pick the rows to contain the 1’s. This gives a total of 9*70 = 630 patterns associated with this partition.The fourth partition contains six 2’s and 3 1’s. There are C(9,6) = (9*8*7*6*5*4)/(6*5*4*3*2*1) = 84 ways to pick the rows which will contain the 2’s. Yet again, there is only one way to pick the rows to contain the 1’s. This gives a total of 84 patterns associated with this partition.The next step is to figure out how to distribute the balls into each of these rows.Any row with a single ball has 10 possible ways to pick that ball.Any row with two balls has C(10,2) = (10*9)/(2*1) = 45 ways to pick them if order of balls in the row doesn’t matter or P(10,2) = 10*9 = 90 ways if the order does matter.Any row with three balls has C(10,3) = (10*9*8)/(3*2*1) = 120 ways to pick them if order doesn’t matter or P(10,3) = 10*9*8 = 720 if the order does matter.The first partition pattern has therefore, 120^3 * 10^6 = 1,728,000,000,000 ways to distribute for each pattern where order doesn’t matter or 720^3 * 10^6 = 373,248,000,000,000 where it does. This yields totals of 145,152,000,000,000 for the 84 patterns where order doesn’t matter or 31,352,832,000,000,000 where it does.The second partition has 120^2*45^2*10^5 = 291,600,000,000 ways to distribute for each pattern where order doesn’t matter or 720^2 * 90^2 * 10^5 = 419,904,000,000,000 where it does. This yields totals of 220,449,600,000,000 for the 756 patterns where order doesn’t matter or 317,447,424,000,000,000 where it does.The third partition has 120*45^4*10^4 = 4,920,750,000,000 ways to distribute for each pattern where order doesn’t matter or 720 * 90^4 * 10^4 = 472,392,000,000,000 where it does. This yields totals of 31,000,725,000,000,000 for the 630 patterns where order doesn’t matter or 297,606,960,000,000,000 where it does.The third partition has 45^6*10^3 = 8,303,765,625,000 ways to distribute for each pattern where order doesn’t matter or 90^6 * 10^3 = 531,441,000,000,000 where it does. This yields totals of 697,516,312,500,000 for the 84 patterns where order doesn’t matter or 44,641,044,000,000,000 where it does.I will let you do the additions yourself. But as another Quoran answered, “a lot!”
Related searches to illinois nfp 104 15 20
Create this form in 5 minutes!
How to create an eSignature for the nfp 104 15 20 assumed corporate
How to make an electronic signature for the Form Nfp 1041520 Rev Aug 2014 Cyberdrive Illinois Cyberdriveillinois online
How to create an eSignature for the Form Nfp 1041520 Rev Aug 2014 Cyberdrive Illinois Cyberdriveillinois in Google Chrome
How to create an eSignature for putting it on the Form Nfp 1041520 Rev Aug 2014 Cyberdrive Illinois Cyberdriveillinois in Gmail
How to create an eSignature for the Form Nfp 1041520 Rev Aug 2014 Cyberdrive Illinois Cyberdriveillinois from your smartphone
How to make an eSignature for the Form Nfp 1041520 Rev Aug 2014 Cyberdrive Illinois Cyberdriveillinois on iOS
How to generate an eSignature for the Form Nfp 1041520 Rev Aug 2014 Cyberdrive Illinois Cyberdriveillinois on Android OS
People also ask nfp 104 15
-
What is nfp 104 15 20, and how does it relate to airSlate SignNow?
NFP 104 15 20 refers to a set of standards for electronic signatures and document management. AirSlate SignNow fully complies with these standards, ensuring that your electronic signatures are legally binding and secure. By utilizing airSlate SignNow, you can manage your documents efficiently while adhering to nfp 104 15 20 regulations.
-
How much does airSlate SignNow cost for users focusing on nfp 104 15 20 compliance?
AirSlate SignNow offers various pricing plans tailored to businesses needing nfp 104 15 20 compliance. Depending on your organization's size and feature requirements, you can select a plan that suits your budget and operational needs. This ensures you benefit from all necessary functionalities while staying within your financial limits.
-
What key features does airSlate SignNow offer for businesses aiming for nfp 104 15 20?
AirSlate SignNow provides a range of features that cater specifically to nfp 104 15 20 requirements, including customizable templates, secure cloud storage, and detailed auditing trails. These features allow you to streamline document workflows while maintaining compliance. Furthermore, the platform enhances collaboration and ensures that all signatures are tracked and managed efficiently.
-
Can I integrate airSlate SignNow with other tools to support nfp 104 15 20 processes?
Absolutely! AirSlate SignNow integrates seamlessly with various third-party applications that support nfp 104 15 20 processes, including CRMs, cloud storage services, and project management tools. This allows you to create a cohesive workflow across different platforms. Integration helps maximize productivity while ensuring adherence to compliance standards.
-
What benefits does airSlate SignNow bring for organizations concerned with nfp 104 15 20?
Using airSlate SignNow allows organizations focused on nfp 104 15 20 to enhance operational efficiency and reduce processing times for documents. Its user-friendly interface streamlines the e-signature process, making it easy to send and sign documents securely. Moreover, it reduces paper usage, contributing to environmentally friendly practices while ensuring compliance.
-
How is data security handled in airSlate SignNow for nfp 104 15 20 compliance?
AirSlate SignNow prioritizes data security through advanced encryption and compliance with nfp 104 15 20 standards. Sensitive document information is protected while in transit and at rest, ensuring that your data remains confidential. Additionally, the platform offers customizable access controls to manage who can view or edit documents.
-
Is training available for using airSlate SignNow to comply with nfp 104 15 20?
Yes, airSlate SignNow provides extensive training resources, including tutorials and customer support, to help users understand how to comply with nfp 104 15 20. These resources are designed to ensure that your team is well-equipped to utilize the platform effectively. This support is crucial for maximizing the benefits of the features and maintaining compliance.
Get more for nfp 104 15 20 adopt cancel
Find out other nfp 105 10
- Can I eSignature Alabama Lawers NDA
- eSignature Wyoming Insurance Stock Certificate Secure
- How To eSignature Wyoming Insurance Stock Certificate
- eSignature Wyoming Insurance Stock Certificate Fast
- How Do I eSignature Wyoming Insurance Stock Certificate
- Help Me With eSignature Wyoming Insurance Stock Certificate
- How Can I eSignature Wyoming Insurance Stock Certificate
- eSignature Tennessee High Tech Cease And Desist Letter Online
- eSignature Wyoming Insurance Stock Certificate Simple
- Can I eSignature Wyoming Insurance Stock Certificate
- eSignature Tennessee High Tech Cease And Desist Letter Computer
- eSignature Wyoming Insurance Stock Certificate Easy
- eSignature Wyoming Insurance Separation Agreement Online
- eSignature Tennessee High Tech Cease And Desist Letter Mobile
- eSignature Wyoming Insurance Separation Agreement Computer
- eSignature Wyoming Insurance Separation Agreement Mobile
- eSignature Wyoming Insurance Stock Certificate Safe
- eSignature Tennessee High Tech Cease And Desist Letter Now
- eSignature Wyoming Insurance Separation Agreement Now
- eSignature Wyoming Insurance Separation Agreement Later