Of 1 FC3104Form 6 Courts Oregon


What is the Form FC 3?
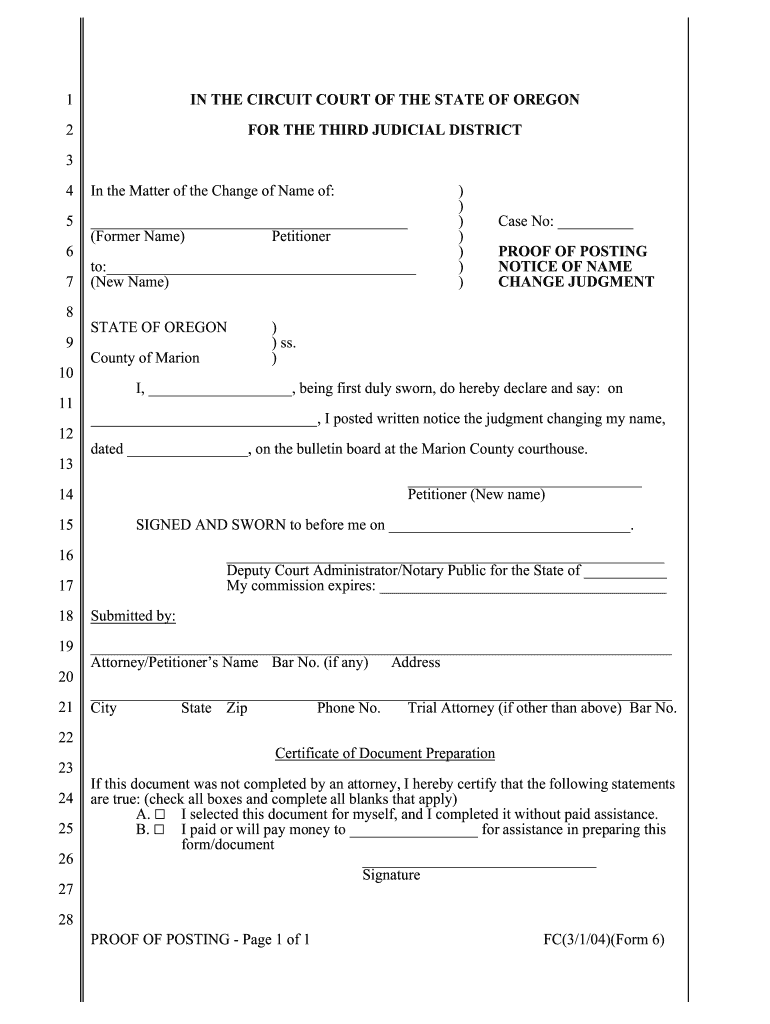
The Form FC 3 is a legal document utilized within the Oregon court system. This form is essential for specific legal proceedings, particularly in family law cases. It serves various purposes, such as initiating a case or providing necessary information to the court regarding the parties involved. Understanding its function is crucial for anyone engaging with the Oregon courts.
How to Use the Form FC 3
Using the Form FC 3 requires careful attention to detail. First, gather all relevant information, including the names and addresses of all parties involved. Next, fill out the form accurately, ensuring that all sections are completed as required. Once filled, the form must be submitted to the appropriate court. It's advisable to keep a copy for personal records.
Steps to Complete the Form FC 3
Completing the Form FC 3 involves several key steps:
- Begin by downloading the form from the official Oregon courts website or obtaining a physical copy from the court.
- Fill in your personal information, including your name, address, and contact details.
- Provide information about the other party involved in the case.
- Complete any additional sections as required by the specific legal context.
- Review the completed form for accuracy before submission.
Legal Use of the Form FC 3
The legal use of the Form FC 3 is governed by Oregon state law. It is crucial that the form is filled out correctly to ensure it is accepted by the court. Failure to comply with legal requirements may result in delays or dismissal of the case. The form must be submitted to the appropriate court for it to have legal standing.
State-Specific Rules for the Form FC 3
Oregon has specific rules regarding the use of the Form FC 3. These rules dictate how the form should be filled out, the information required, and the submission process. It's important for users to familiarize themselves with these regulations to ensure compliance and avoid potential legal issues.
Who Issues the Form FC 3
The Form FC 3 is issued by the Oregon judicial system. It is specifically designed for use in family law cases and is available through the official Oregon courts website. Users should ensure they are using the most current version of the form to meet legal standards.
Quick guide on how to complete form fc 3
Complete form fc 3 effortlessly on any device
Online document management has gained popularity among organizations and individuals. It offers an ideal eco-friendly alternative to traditional printed and signed documents, as you can locate the appropriate form and securely store it online. airSlate SignNow equips you with all the necessary tools to create, modify, and electronically sign your documents swiftly without delays. Manage form fc 3 on any platform using airSlate SignNow Android or iOS apps and simplify any document-related task today.
The easiest way to edit and electronically sign oregon courts with ease
- Locate form fc 3 and click on Get Form to begin.
- Utilize the tools we provide to complete your document.
- Highlight important sections of your documents or conceal sensitive information using tools specifically offered by airSlate SignNow for that purpose.
- Create your eSignature with the Sign tool, which takes mere seconds and carries the same legal validity as a conventional wet ink signature.
- Review all the details and click on the Done button to save your changes.
- Choose how you wish to deliver your form, by email, SMS, or invitation link, or download it to your computer.
Eliminate concerns over lost or misplaced files, tedious form searches, or errors that necessitate printing new document copies. airSlate SignNow satisfies all your requirements for document management in just a few clicks from any device of your preference. Edit and electronically sign oregon courts and ensure effective communication at every stage of the form preparation process with airSlate SignNow.
Create this form in 5 minutes or less
FAQs oregon courts
-
Mathematical Puzzles: What is () + () + () = 30 using 1,3,5,7,9,11,13,15?
My question had been merged with another one and as a result, I have added the previous answer to the present one. Hopefully this provides a clearer explanation. Just using the numbers given there, it's not possible, because odd + odd = even, even + odd = odd. 30 is an even number, the answer of 3 odd numbers must be odd, it's a contradiction. If what people say is true, then the question is wrongly phrased its any number of operations within those three brackets must lead to 30. Then it becomes a lot easier. Such as 15 + 7 + (7 + 1). That would give 30. But it assumes something that the question does not state explicitly and cannot be done that way. I still stick to my first point, it can't be done within the realm of math and just using three numbers, if not, then the latter is a way to solve it.EDIT: This question has come up many times, Any odd number can be expressed as the following, Let [math]n, m, p[/math] be an odd number, [math] n = 1 (mod[/math] [math]2), m = 1 (mod[/math] [math]2), p = 1 (mod[/math] [math]2)[/math][math]n+m+p = 1 + 1 + 1 (mod[/math] [math]2)[/math]Let's call [math]n+m+p[/math] as [math]x[/math][math]=> x = 3 (mod[/math] [math]2)[/math]Numbers in modulo n can be added, I'll write a small proof for it below, [math]a = b (mod[/math] [math]n), c = d (mod[/math] [math]n)[/math][math]a+c = b+d (mod[/math] [math]n)[/math]We can rewrite [math]b[/math] and [math]d[/math] in the following way, [math]n | (b - a) => b-a = n*p[/math] (for some integer p) [math]b = a + np[/math][math]b = a + np, d = c + nq[/math][math]b + d = a + np + c + nq[/math][math]b+d = a + c + n(p + q)[/math]Now we have shown that our result is true, moving forward, [math]3 = 1 (mod[/math] [math]2)[/math][math]x = 1 (mod[/math] [math]2)[/math]Therefore the sum of three odd numbers can never be even. It will always be congruent to 1 in mod 2.(This was what I wrote for a merged answer).Modular arithmetic - Link on modular arithmetic, the basic operations. Modular multiplicative inverse - The multiplicative inverse in modular operations.Congruence relationFermat's little theorem Modular exponentiation - As title suggests.Good luck!
-
How much liquid required to fill the tank if the tank was 1/3 portion full, after 50 litre liquid drain out it remains 1/6 of the tank?
TANK CAPACITY = 6 X 50 = 300INITIALLY IT IS (2.3) FREE i.e 200 LITRESREQUIRED LIQUID = 200 LITRES
-
How can one make 10,000 out of the digits 1, 2, 3, 4, 5, 6, 7, 8, and 9, in order?
[math](1\!+\!2\!+\!3\!+\!4)^{5+6-7} \cdot (-8\!+\!9) = 10000[/math]
-
Is every prime number other than 2 and 3 of the form (6k±1)? Is this a proven result? What are other resources about it?
Every integer can be written in the form of [math]6n,6n+1,6n+2,6n+3,6n+4[/math] or [math]6n+5[/math].It is quite evident that [math]6n[/math] is always divisible by 6, 6n+1 may be prime, [math]6n+2[/math] will always be divisible by 2, [math]6n+3[/math] by 3, and [math]6n+4[/math] by 2. [math]6n+5[/math] may be prime. Our candidates for primality are therefore [math]6n+1[/math] and [math]6n+5[/math]. We can then see that [math]6n+5=6(n+1)-1=6x-1[/math] Therefore, every prime can be written in the form of [math]6n \pm 1[/math]
-
Five-digit numbers are formed using the digits 1, 2, 3, 4, 5, 6. Out of these, how many are even?
720 Five-digit numbers will be formed using the digits 1,2, 3,4,5,6. Out of them 360 Five -digit numbers will be even. You can do the math-If we place the 2,4,6 at one’s place the digits formed will be even_ _ _ _ 2Now total no.of ways to arrange 4 numbers out of 5 numbers will be 5! Which is 120Similarly when 4,6 are placed at one’s place, total no.of arrangements are 120 and 120So this gives 360 arrangements.There will be 360 such arrangements which will be even.Thanks for reading my answer i hope you have understand.
-
What is the algorithm to find all the solutions of making 100 out of 1-2-3-4-5-6-7-8-9 in order?
It's a classic textbook example of (simple) recursion.(However there's no pruning heuristic to allow early rejection of invalid candidates, since due to all the operations e.g. /, % and √ (and boolean operations like | & ^ and C operations like ~ you can't know if something is way too high/too low/not divisible.So to give a ballpark estimate, if there are B binary operators (neglect unary operators like √ ~ !), and we have to choose one of those to connect the N=9 digits strictly in order with N-1 operators, we must evaluate all (N-1)^B candidates. So for + - * / % that's already 8^5 = 2^15 candidates.If you allow parentheses on two or more adjacent terms there are exponentially more options. I can't enumerate those off the top of my head but let's say there are T terms.And then further if you consider there are U unary operators (e.g. √ ~ ! and maybe unary reductions operators like ^ & |), and if we estimate by assuming that each of the T terms (i.e. either number or parenthesized subexpression) can optionally have one of those U operators applied once, this multiplies the number of candidates by a further factor T*U
-
How many 3 digit numbers can be formed using the digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 if repetitions of digits are allowed?
This question can be answered using two methods. Let's start with the simplest one.Method 1:The number is three digits, so for them let's take three blanks _ _ _The first blank can be filled using any of the digits from 1–9 because if we use zero to fill the first blank the number becomes of two digits. Hence we have 9 ways to fill the first blank.Now, the second blank can be filled by any of the remaining 10 digits because repetition is allowed and thus the digit selected for the first blank can also be selected. So 10 ways.Similarly 10 ways for the third blank.So total number of combinations become 9 x 10 x 10 = 900Hence the answer is 900 such number can be formed.Method 2:Since the first digit cannot be zero, we have 9C1 ways to select the first digit (one digit selected from a set of nine distinct digits). (9C1 = 9)Now, for the remaining two places we can have zero as well. Hence we have 10C1 ways to select a digit for tens and ones place each. (10C1 = 10)Hence total number of combinations become 9C1 x 10C1 x 10C1 = 9 x 10 x 10 = 900Hence the answer is 900 such numbers can be formed.Hope it helped you! :)
Related searches to form fc 3
Create this form in 5 minutes!
How to create an eSignature for the oregon courts
How to create an eSignature for your Of 1 Fc3104form 6 Courts Oregon online
How to generate an electronic signature for the Of 1 Fc3104form 6 Courts Oregon in Chrome
How to create an electronic signature for putting it on the Of 1 Fc3104form 6 Courts Oregon in Gmail
How to create an eSignature for the Of 1 Fc3104form 6 Courts Oregon from your mobile device
How to make an electronic signature for the Of 1 Fc3104form 6 Courts Oregon on iOS
How to create an eSignature for the Of 1 Fc3104form 6 Courts Oregon on Android devices
People also ask oregon courts
-
What is the form FC 3, and how does it work?
The form FC 3 is a crucial document for businesses that require efficient management and signing of contracts and agreements. With airSlate SignNow, you can easily create, send, and eSign your form FC 3, streamlining your workflow and reducing paper clutter.
-
How much does it cost to use airSlate SignNow for form FC 3?
airSlate SignNow offers competitive pricing plans tailored to different business needs. You can access all features, including the management of form FC 3, at an affordable monthly fee, ensuring you get the best value for your investment.
-
What features does airSlate SignNow offer for form FC 3?
airSlate SignNow equips you with a range of features specifically designed to enhance your experience with form FC 3. These include easy document creation, customizable templates, automated workflows, and secure eSignature options, making it a comprehensive solution.
-
How can using form FC 3 improve our business efficiency?
Utilizing form FC 3 with airSlate SignNow can signNowly enhance your business efficiency by automating the document management process. This means faster turnaround times, reduced errors, and an overall streamlined process that saves you time and resources.
-
Can I integrate airSlate SignNow with other tools for managing form FC 3?
Yes, airSlate SignNow seamlessly integrates with a variety of third-party applications, allowing you to manage form FC 3 alongside other tools you may already be using. This integration helps you maintain new workflows and ensures all your documentation needs are met efficiently.
-
Is airSlate SignNow secure for handling sensitive form FC 3 documents?
Absolutely! airSlate SignNow prioritizes security by implementing advanced encryption protocols and compliance with industry standards. This ensures that your form FC 3 and all documents remain confidential and secure throughout the eSigning process.
-
What are the benefits of using airSlate SignNow for form FC 3 over traditional methods?
The primary benefit of using airSlate SignNow for form FC 3 is the signNow reduction in processing time compared to traditional paper methods. Additionally, it minimizes the risk of lost documents and provides a user-friendly interface that makes the signing process quick and easy.
Get more for form fc 3
- Change report form final department of public health and social dphss guam
- Nhjb 2065 f form
- Consent form singapore general hospital
- Standard permissionmedical release form the united church uccmedfield
- Ir 25 form 2016
- Kooloaula housing application form
- Dc unemployment form
- Amway member ship fom 2016 pdf form
Find out other oregon courts
- How To eSignature Montana Police Medical History
- eSignature Montana Police Medical History Computer
- eSignature Montana Police Medical History Mobile
- eSignature Nevada Police Letter Of Intent Online
- How Do I eSignature Montana Police Medical History
- eSignature Nevada Police Letter Of Intent Computer
- eSignature Montana Police Medical History Now
- eSignature Nevada Police Letter Of Intent Mobile
- eSignature Nevada Police Letter Of Intent Now
- Help Me With eSignature Montana Police Medical History
- eSignature Nevada Police Letter Of Intent Later
- eSignature Montana Police Medical History Later
- eSignature Nevada Police Letter Of Intent Myself
- eSignature Nevada Police Letter Of Intent Free
- eSignature Nevada Police Letter Of Intent Secure
- eSignature Montana Police Medical History Myself
- eSignature Nevada Police Letter Of Intent Fast
- How Can I eSignature Montana Police Medical History
- eSignature Montana Police Medical History Free
- eSignature Nevada Police Letter Of Intent Simple