Establishing secure connection…Loading editor…Preparing document…
We are not affiliated with any brand or entity on this form.
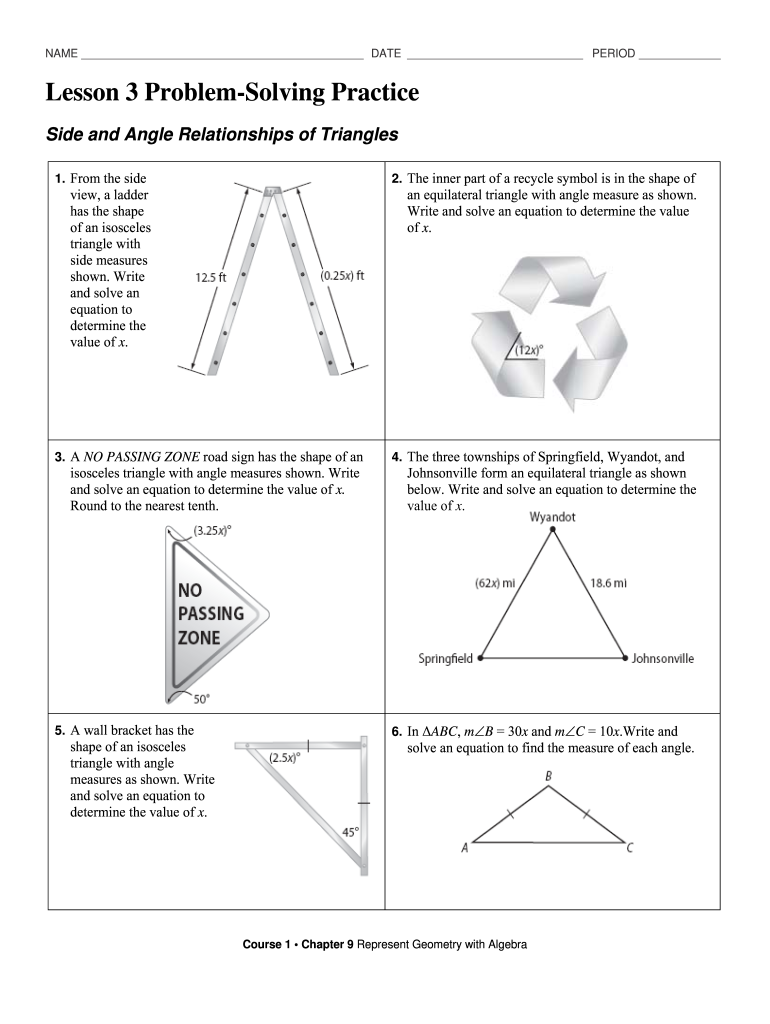
Lesson 3 Problem Solving Practice Angles of Triangles Answer Key Form
Understanding the Lesson 3 Problem Solving Practice Angles of Triangles Answer Key
The Lesson 3 Problem Solving Practice Angles of Triangles Answer Key provides essential solutions for students working on angle relationships in triangles. This answer key serves as a reference tool, allowing learners to verify their work and understand the reasoning behind each solution. It covers various types of problems related to angles, including complementary and supplementary angles, as well as the properties of triangles. By using this resource, students can enhance their comprehension of geometric principles and improve their problem-solving skills.
How to Effectively Use the Lesson 3 Problem Solving Practice Angles of Triangles Answer Key
To maximize the benefits of the Lesson 3 Problem Solving Practice Angles of Triangles Answer Key, students should first attempt to solve the problems independently. After completing the exercises, they can refer to the answer key to check their answers. It is important to not only look at the final answers but also to review the methods used to arrive at those solutions. This practice reinforces learning and helps identify areas where further study may be needed. Additionally, discussing the answers with peers or teachers can provide deeper insights into the concepts involved.
Obtaining the Lesson 3 Problem Solving Practice Angles of Triangles Answer Key
The Lesson 3 Problem Solving Practice Angles of Triangles Answer Key can typically be found through educational resources provided by schools or online educational platforms. Students should check with their teachers for any official distributions or access points. In some cases, answer keys may be included in textbooks or supplementary materials. For online resources, ensure that the site is reputable and aligns with educational standards to guarantee the accuracy of the content.
Steps to Complete the Lesson 3 Problem Solving Practice Angles of Triangles Exercises
Completing the Lesson 3 Problem Solving Practice Angles of Triangles exercises involves several key steps:
- Read the instructions carefully to understand the requirements of each problem.
- Identify the types of angles and triangles involved in each question.
- Apply relevant geometric principles and theorems to find solutions.
- Double-check calculations to ensure accuracy.
- Use the answer key to verify your solutions and understand any discrepancies.
Key Elements of the Lesson 3 Problem Solving Practice Angles of Triangles Answer Key
Key elements of the Lesson 3 Problem Solving Practice Angles of Triangles Answer Key include:
- Clear and concise solutions to each problem.
- Explanations for the reasoning behind each answer.
- Examples of similar problems to reinforce learning.
- Visual aids, such as diagrams, to illustrate concepts effectively.
Legal Considerations for Using the Lesson 3 Problem Solving Practice Angles of Triangles Answer Key
When using the Lesson 3 Problem Solving Practice Angles of Triangles Answer Key, it is important to ensure that the material is used in compliance with copyright laws. Educational resources often have specific guidelines regarding their use in classrooms or for personal study. Always attribute the source of the answer key if required, and avoid distributing it without permission. This respect for intellectual property fosters a fair educational environment and encourages the creation of quality resources.
Quick guide on how to complete angle problem solving
The simplest method to locate and approve angle problem solving
On the scale of your entire organization, ineffective procedures surrounding document approval can consume signNow amounts of productive time. Authorizing documents such as lesson 3 homework practice angles of triangles is an inherent aspect of operations in any enterprise, which is why the effectiveness of each agreement’s lifecycle greatly impacts the organization’s overall success. With airSlate SignNow, endorsing your lesson 3 homework practice triangles is as straightforward and swift as possible. This platform provides you with the latest version of virtually any form. Even better, you can approve it immediately without needing to download external applications on your device or printing physical copies.
How to obtain and approve your lesson 3 problem solving practice answer key
- Browse our collection by category or utilize the search bar to locate the document you require.
- View the form preview by selecting Learn more to verify it is the correct one.
- Select Get form to start editing right away.
- Fill out your form and incorporate any necessary details using the toolbar.
- Upon completion, click the Sign tool to endorse your answer key my homework lesson 3.
- Pick the signature method that suits you best: Draw, Create initials, or upload an image of your handwritten signature.
- Click Done to finalize editing and proceed to document-sharing options if required.
With airSlate SignNow, you possess all the tools necessary to handle your documentation efficiently. You can discover, complete, modify and even send your [SKS] in a single tab with no complications. Enhance your workflows with a singular, intelligent eSignature solution.
be ready to get more
Create this form in 5 minutes or less
Video instructions and help with filling out and completing Lesson 3 Problem Solving Practice Angles Of Triangles Answer Key Form
Instructions and help about angle relationships answer key
FAQs chapter 3 angles and triangles answer key
-
How do I write pseudo code for this: "input 3 side of a triangle and figure out if its scalene, isosceles, equilateral or right angle triangle?
I'm not gonna give you that exact pseudo code here, instead let's break down problem . There is two option to define any side of Triangle (taking input as well)... a. As Cartesian (or Polar) Coordinate of the intersecting point of any two adjacent sides b. Defining Each Sides with Equation. Lets start with easier one... (taking input as coordinate of three intersection point of adjacent sides of course)Once you have have all three points you can measure the length of each sides.Lucky in Triangle all sides are adjacent to others (that make the calculation whole lot easier). Use following Equation to find the length of each sides from their intersecting coordinates. r= √( (x1-x2)^2+ (y1 - y2)^2), where x1 adjacent to x2 and so on... & r is length of each side.Once you get all sides measured you're good to go for final stage...1. if all sides are same in length (which is a good news!) , the triangle is Equilateral and nothing else (that match your other criteria). Because, No Equilateral could be Right Angle. Wonder Why? Try recalling Pythagoras Theorem about Right angle Triangle. 2. Scalene, Isosceles both can be Right Angle. How to figure out if a triangle is Right Angle, That Pythagoras dude! hypotenuse^2 = adjacent^2 + opposite^2
-
How do I create a fillable HTML form online that can be downloaded as a PDF? I have made a framework for problem solving and would like to give people access to an online unfilled form that can be filled out and downloaded filled out.
Create PDF Form that will be used for download and convert it to HTML Form for viewing on your website.However there’s a lot of PDF to HTML converters not many can properly convert PDF Form including form fields. If you plan to use some calculations or validations it’s even harder to find one. Try PDFix Form Converter which works fine to me.
-
The perimeter of a right angled triangle is 72 cm, and the lengths of its sides are in the ratio 3:4:5. How do you work out the area?
The perimeter of a right angled triangle is 72 cm, and the lengths of its sides are in the ratio 3:4:5. How do you work out the area?Begin by working out the length of the three sides.Because the sides are in a ratio of 3:4:5 and the perimeter is 723x + 4x + 5x = 72 : combine like terms12x = 72 : isolate x by dividing both sides by 12x =6The sides are 18 cm, 24 cm and 30 cm.In a right triangle the two shorter sides are always perpendicular, so the area of any right triangle is: A = (1/2) • s₁ • s₂ where s₁ and s₂ are the two shorter sides.A = (1/2) • 18 cm • 24 cm = 216 cm²
-
How do you solve a mathematical question if you are given all the sides of a triangle and you are asked to look for one angle?
You can use the cosine rule for a triangle.cos(A) = (b^2 + c^2 - a^2)/2bcSimilarly for,cos(B) = (a^2 + c^2 - b^2)/2accos(C) = (b^2 + a^2 - c^2)/2ab
-
How does one formulaically determine the precise angle to bend the four sides of a given sheet metal pyramid? I have worked this out using projection drawings and trigonometry, but wonder if anyone has one formula to solve a variety of dimensions?
I assume that you have in mind a pyramid with a square base and four identical triangular sides that rise symmetrically to a common vertex located directly above the center of the base. With apologies for the poor artwork, let [math]H[/math] and [math]W[/math] be the pyramid’s height and base width, respectively, and let [math]\theta[/math] be the angle of inclination of the sides relative to the base:Then[math]\ \cos \theta = \frac{W/2}{\sqrt{(W/2)^2 + H^2}},\ [/math]so that the angle itself is the inverse cosine of that quantity:[math]\ \theta = \arccos \left ( \frac{1}{\sqrt{1 + 4(H^2/W^2)}}\right )\ [/math](Editing to add the computation of a different angle - the one between neighboring sides)You can express the angle between neighboring sides as the angle between the normal (perpendicular) vectors to the respective sides.Consider a coordinate system in which the axes point in the following directions:1) out from the center of the base of the pyramid to the midpoint of the bottom of the first side of interest;2) vertically, from the center of the base up through the vertex;3) out from the center of the base to the midpoint of the second side of interest.A normal vector to the first side can be written as [math](H, W/2, 0)[/math], and a normal vector to the second side as [math](0, W/2, H)[/math]. If \alpha is the angle between these vectors, then:[math]\ \cos \alpha = \frac{(H, W/2, 0) \cdot (H, W/2, 0)}{|(H, W/2, 0)| |(H, W/2, 0)|},\ [/math]so that the angle between the sides is:[math]\ \alpha = \arccos \left ( \frac{1}{1 + \frac{4H^2}{W^2}} \right )\ [/math]
-
Is it good practice to watch YouTube videos and Khan Academy to learn how to solve problems in engineering school, instead of figuring them out yourself?
Yes of course. The more you go through materials the more you learn. Instead of figuring the solutions yourself you can use SolutionInn - Online Tutoring | Get Study Help and Textbook Solutions for solved textbook solutions.
-
How can we solve this question? A square of a side x cm has the same area as a rectangle of length (3x+5) cm and width (2x-3). How can you form an equation in x? How can you show that it simplifies to 5x+x-15?
How can we solve this question? A square of a side x cm has the same area as a rectangle of length (3x+5) cm and width (2x-3). How can you form an equation in x? How can you show that it simplifies to 5x+x-15?Q1: What is the area of a square?A1: The length of its side squaredSo, the area of the square in your question (in square centimetres) [math]= x^2[/math]Q2: What is the area of a rectangle?A2: The length of its long side multiplied by the length of its short side.So, the area of the rectangle in your question (in square centimetres) [math]= (3x + 5)(2x - 3) = 6x^2 + x - 15[/math]From the question, we are told that the area of the square is the same as the area of the rectangle, so:[math]x^2 = 6x^2 + x - 15[/math]Subtracting [math]x^2[/math] from both sides of the equation, we have:[math]5x^2 + x - 15 = 0[/math][math]\\[/math]We now need to solve this quadratic equation, i.e. find the values of [math]x[/math] such that the equation is true. To help us, there is a simple formula we can use.The solution to the general quadratic [math]ax^2 = bx + c = 0[/math] is:[math]x = \frac {-b \pm \sqrt{b^2 - 4ac}}{2}[/math]For our equation, we have [math]a = 5[/math], [math]b = 1[/math] and [math]c = -15[/math]Slotting these values into our formula, we have:[math]x = \frac {-1 \pm \sqrt{1^2 - 4 \ times 5 \times 15}}{2 \times 10} = \frac {-1 \pm \sqrt{1 + 300}}{10}[/math][math]= \frac {-1 \pm \sqrt{301}}{10}[/math]Well, its clear that we can;’t have a negative length, so the answer is:[math]x = \sqrt {3.01} - 0.1 \approx 1.634935\ cm[/math]
Related searches to course 3 chapter 5 triangles and the pythagorean theorem answer key lesson 1 homework practice
Glencoe Geometry Chapter 3 Test Form 2b answer key
Glencoe Geometry Chapter 3 Test answer key
Create this form in 5 minutes!
How to create an eSignature for the course 3 chapter 5 triangles and the pythagorean theorem lesson 3 skills practice
How to make an electronic signature for the Lesson 3 Problem Solving Practice Side And Angle Relationships Of Triangles Form in the online mode
How to create an electronic signature for the Lesson 3 Problem Solving Practice Side And Angle Relationships Of Triangles Form in Chrome
How to make an eSignature for putting it on the Lesson 3 Problem Solving Practice Side And Angle Relationships Of Triangles Form in Gmail
How to make an eSignature for the Lesson 3 Problem Solving Practice Side And Angle Relationships Of Triangles Form straight from your mobile device
How to make an eSignature for the Lesson 3 Problem Solving Practice Side And Angle Relationships Of Triangles Form on iOS devices
How to generate an electronic signature for the Lesson 3 Problem Solving Practice Side And Angle Relationships Of Triangles Form on Android devices
People also ask angle key
-
What is the lesson 3 extra practice angles of triangles answer key?
The lesson 3 extra practice angles of triangles answer key is a comprehensive guide that provides detailed solutions and explanations for triangle angle problems. This resource is perfect for students and educators who seek clarity and understanding regarding triangle properties and relationships.
-
How can I access the lesson 3 extra practice angles of triangles answer key?
You can access the lesson 3 extra practice angles of triangles answer key through our online platform. By signing up for a subscription, you'll receive instant access to this answer key along with other educational resources that enhance learning and teaching.
-
Is there a cost associated with the lesson 3 extra practice angles of triangles answer key?
Yes, there is a subscription fee for accessing the lesson 3 extra practice angles of triangles answer key. However, our pricing is designed to be cost-effective, providing value through a wealth of educational content and tools that further aid in understanding geometry.
-
What features come with the lesson 3 extra practice angles of triangles answer key?
The lesson 3 extra practice angles of triangles answer key includes step-by-step solutions, visual aids, and practice exercises. Additionally, it is integrated with interactive tools that allow users to explore angles and triangles in a practical, engaging way.
-
How can the lesson 3 extra practice angles of triangles answer key benefit students?
The lesson 3 extra practice angles of triangles answer key benefits students by providing clarity on complex concepts through clear explanations and examples. It enhances their understanding of triangles, which is crucial for their success in geometry and related subjects.
-
Can the lesson 3 extra practice angles of triangles answer key be integrated with other educational tools?
Yes, the lesson 3 extra practice angles of triangles answer key is designed to work seamlessly with other educational tools and platforms. This integration allows for a more interactive learning experience, making it easier for educators to incorporate it into their lesson plans.
-
What age group is the lesson 3 extra practice angles of triangles answer key suitable for?
The lesson 3 extra practice angles of triangles answer key is suitable for middle to high school students, typically ranging from grades 6 to 10. It's crafted to align with educational standards for geometry, ensuring that learners grasp essential triangle concepts.
Get more for using angle relationships to find angle measures worksheet answers
- Mela permission letter form
- Telepay format
- Trust deed sample pakistan form
- Sample job application rphsbusinessorg form
- Quicklink to expense reimbursement form stjoronk
- Aktiebok mall gratis form
- Modified july government of pakistan planning commission pc1 form social sectors 1 name of the project strengthening of
- Electrical hours verification form 100072122
Find out other angle relationships in triangles
- Electronic signature Vermont NDA Later
- Electronic signature Vermont NDA Myself
- Electronic signature Virginia NDA Now
- Electronic signature Vermont NDA Free
- eSign South Dakota Online Donation Form Online
- Electronic signature Vermont NDA Secure
- Electronic signature Virginia NDA Later
- Electronic signature Vermont NDA Fast
- Electronic signature Vermont NDA Simple
- Electronic signature Vermont NDA Easy
- eSign South Dakota Online Donation Form Computer
- Electronic signature Virginia NDA Myself
- Electronic signature Vermont NDA Safe
- Electronic signature Virginia NDA Free
- eSign South Dakota Online Donation Form Mobile
- How To Electronic signature Virginia NDA
- Electronic signature Virginia NDA Secure
- How Do I Electronic signature Virginia NDA
- Help Me With Electronic signature Virginia NDA
- How Can I Electronic signature Virginia NDA
be ready to get more
Get this form now!
If you believe that this page should be taken down, please follow our DMCA take down process here.