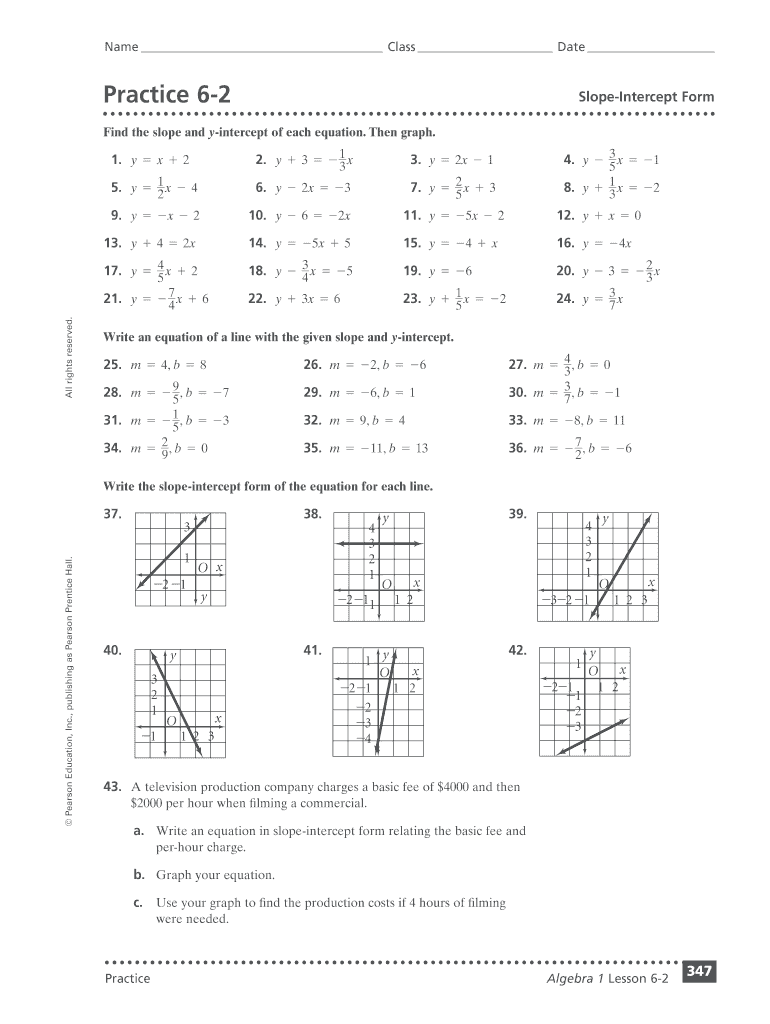
Practice 6 2 Slope Intercept Form Answer Key

I prefer to discuss linear equations in terms of real-world situations since you encounter them so often.Suppose you sign up for a phone service. The phone costs $400, the activation fee is $100, and the plan costs $60 per month.How much do you pay? (What is y?)It depends. (That’s why they call it a dependent variable.)Depends on what?It depends on the number of months: x.y = mx +btotal cost =($60 per month)(# of months) + $100 + $400y = 60x + 500So let’s just take the x out.y = 60 + 500y = 560So, even if you use your phone until the end of time, your total cost comes out to $560. That’s because your monthly bill is now being multiplied by 1, rather than a variable. Your bill (y) is no longer dependent on how many months you use it (x). You pay your “monthly” bill once.How does that affect the graph?Instead of your bill increasing as the number of months increases, it doesn’t.Your slope is now 0.y = 560 = 0x + 560

The line [math] y = 1 [/math] is a horizontal line on the [math] x,y [/math] plane. The slope is 0.​​Since we want our function to pass through the point [math](2,-1)[/math] then we'll have to choose our [math] y[/math] value of our point to form our function. ​​

There are two steps to writing these equations.Find the Slope Fraction for the line.(This is the “m” in y=mx+b).Figure out how far up or down you have to move the line to match the two points.(This is the +b part of y=mx+b).Find the slope fraction from the line:There are two ways to find the slope from two points.The visual way is to graph each point and then count the number of squares up (rise), then the number of squares over (run) between the points.This makes the slope fraction [math]\frac{rise}{run}[/math]Graphing the two points in a.) gives me a rise of 3 and a run of 2. The slope fraction is 3/2.The other way to find the slope is with Arithmetic. You subtract one Y value from the other and one X value from the other. This makes the slope fraction [math]\frac{(Y_1-Y_2)}{(X_1-X_2)}[/math]Using arithmetic for the points in b.) gives me [math]\frac{(5–8)}{(-3 - (-6))}[/math]Doing the subtraction[math]\frac{(-3)}{(3)}[/math]and reducing the fraction gives me[math]\frac{(-1)}{(1)}[/math]Which reduces even further to -1. -1 is the “slope fraction” for the second set of points.Move the line up or down with +bAfter you know the slope fraction, the next part is to figure out the “b” part of y=mx+b. Here’s how.Visually:Take a piece of graph paper and graph the two points you are given.Now take the slope fraction you found before, and plug it into this equation. y=(slope fraction) * x and graph it.If your line and the two points line up perfectly, then you are done. The equation for the line is y=(slope fraction) * x. That is the case for your first set of points. The answer is [math]y=\frac{3}{2}x[/math]With many problems, the line and your two target points won’t line up. There will be a gap between them. You have to move your y=(slope fraction) * x line up or down to make them line up.To do this, count how far above or below the points your line is. If your line is below the points, add this number to your y=(slope fraction) * x equation. If your line is below the points, subtract the number from your y=(slope fraction) * x equation.This next part is very important. Once you have your y=(slope fraction) * x + (some number) equation, graph it and make sure your answer is correct. If it isn’t, keep playing with the line, moving it up or down, until you find the right offset.You can also find this move-up-or-down number with arithmetic. Here’s how.Take one of your points (x, y) and your slope fraction (slope fraction is really long to type. Let’s call this M instead of “slope fraction”) , and plug it in this equation.b = y - mx(If you look closely at this, you’ll see I just rearranged “y=mx+b” to make this.)Once you’ve figured out b, you can put it in the y=mx+b equation and have your answer.Here’s what this looks like for your problem b.)We know the slope fraction = m = -1.We know one of the points is (-3,5).Plugging that in to my b=y-mx equation looks like this.b = 5 - (-1)*-3.Simplifying…b= 5- 3b= 2… and now I can plug the slope fraction (m) and this value for b into y=mx+b.y=mx+by= (-1)x + 2y= -x + 2Last but not least, and most importantly, I want to check this answer to make sure it’s right. I can do this by graphing the equation to make sure the points line up, or by plugging in the known points to make sure they work in the equationFrom problem b, the points are(-3, 5)plugging this in to y=-x+2 gives 5=- (-3) +2.This simplifies to 5=5.5 is equal to 5, so this point checks out.(-6, 8)plugging this in to y=-x+2 gives 8=- (-6) +2.This simplifies to 8=88 is equal to 8, so this point checks out.Both points check, so y=-x+2 is the answer to question b.)HTH

The line passing through (-1, 2) and (3, 1) goes down 1 (1–2 = -1) as it goes over 4 (3 -(-1) = 4), so it has slope -1/4. The perpendicular to a line of slope m has slope -1/m, so the perpendicular line has slope 4 (= -1/(-1/4)). So the line you are looking for has equationy = 4x+2

To start, let’s look at the general setup of equations in slope-intercept form.(y-y1)=m(x-x1), with m being the slope, and x1 and y1 being the coordinates of the given point on the graph. Now, all you have to do is plug in the information where it belongs.The y coordinate replacing y1, the x coordinate replacing x1, and the slope replacing m.The equation of the line is y= -5, meaning the slope is zero. So for m, you would plug in zero.Equation: y-(-5)=0(x-(-5)y+5=0(x+5)Final: y+5=0

We know:[math]-6m+c=5[/math]And we know:[math]mx+c \ne -12+4x[/math]We can solve this like a normal equation:[math]x \ne \frac{-(12+c)}{m-4}[/math]But for any [math]m[/math] we enter we will get a vaild [math]x[/math], except for the case [math]m=4[/math]So we have the form[math]4x+c[/math]And from there we know:[math]-24+c=5[/math]we add [math]24[/math] to both sides and get:[math]c=29[/math]So our line has the form [math]4x+29[/math]And it it easy to see that it is paralell since [math]-12 \ne 29[/math].

Couple of things you could do. There are plenty of ways to find slope and intercept, but here are two:(From what you have said, I am assuming you are starting with standard form)Ax + By = Cy-intercept is C/B (0, C/B)x-intercept is C/A (C/A, 0)(these are derived from inputting 0 as X or Y)You can plot these and use them to determine a slope:(C/B - 0)/(0 - C/A)= C/B * A/-C= -A/BOr you can go straight to converting the equation:Ax + By = CBy = -Ax + Cy = (-A/B)x + (C/B)from this equation, you can easily see the slope and intercept.Hope this helped!

By putting the given line 3x+2y = 16 in slop form y=mx+c, we have, y = (-3/2)x + 8. Hence the slope of this line is -3/2. Any line perpendicular to it will have the slope equal to 2/3 as the product of slopes of two mutually perpendicular lines is -1.Since this desired line having slope 2/3 passes through(9,-2), its equation will be given by y-y1 = m(x-x1), i.e., y+2 = (2/3) (x-9), i.e. 3y+6 = 2x-18, i.e., 2x-3y = 24. Its intercept form will be obtained by dividing the equation by 24 (the constant term) on both the sides. We shall have: (x/12) + (y/-8) = 1. Means the line makes intercept of 12 on positive X axis, and of 8 on negative Y axis.

Form W-2 is an obligatory form to be completed by every employer. Form W-2 doesn’t have to be filled out by the employee. It is given to inform the employee about the amount of his annual income and taxes withheld from it.You can find a lot of information here: http://bit.ly/2NjjlJi

The y mx b worksheet answer key provides the solutions to problems involving the slope-intercept form of a linear equation. It is a valuable resource for students and educators, aiding in the understanding of algebraic concepts. Utilizing this worksheet can enhance your skill in solving equations efficiently.

airSlate SignNow streamlines your document signing process, allowing you to send and eSign documents quickly and efficiently. With a user-friendly interface and robust features, it empowers businesses to manage documents while saving time and resources. This efficiency is essential for handling documents like the y mx b worksheet answer key seamlessly.

Yes, airSlate SignNow offers a free trial, allowing you to explore its features and benefits without any commitment. This trial includes access to all the functionalities necessary to manage document workflows, including the ability to eSign documents using tools similar to those used for the y mx b worksheet answer key.

airSlate SignNow provides various pricing plans tailored for different business needs, making it a cost-effective solution. Each plan includes essential features for sending and eSigning documents, ensuring that you can find a budget-friendly option that meets your requirements. This flexibility is beneficial for those who need to manage documents like the y mx b worksheet answer key.

Absolutely! airSlate SignNow seamlessly integrates with various software applications, enhancing your workflow. Whether you use CRM systems or document management tools, these integrations facilitate easy access to your documents, including any related to the y mx b worksheet answer key.

Security is a top priority for airSlate SignNow, which offers features such as encrypted document storage and secure electronic signatures. This ensures that your documents, including those involving sensitive information like the y mx b worksheet answer key, are protected against unauthorized access and data bsignNowes.

airSlate SignNow enhances collaboration by enabling multiple users to review, comment, and sign documents simultaneously. This real-time collaboration feature promotes efficient teamwork, making it easier to work together on assignments like the y mx b worksheet answer key. You can keep everyone in sync, leading to quicker approvals.