1 Worksheet Characteristics of Parabolas Form


What is the 1 Worksheet Characteristics Of Parabolas
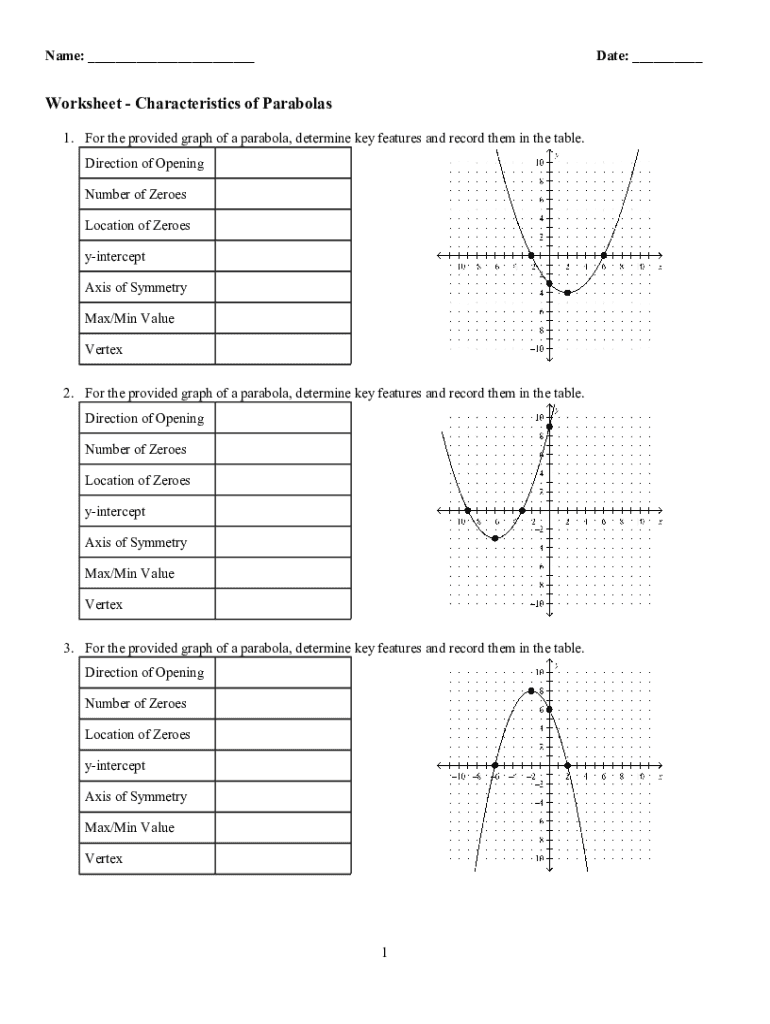
The 1 Worksheet Characteristics Of Parabolas is an educational resource designed to help students understand the fundamental properties and features of parabolas. This worksheet typically includes various exercises that focus on identifying key characteristics such as the vertex, axis of symmetry, direction of opening, and the focus and directrix of the parabola. It serves as a practical tool for reinforcing concepts learned in algebra and geometry, allowing students to apply their knowledge in a structured format.
How to use the 1 Worksheet Characteristics Of Parabolas
To effectively use the 1 Worksheet Characteristics Of Parabolas, students should first review the relevant mathematical concepts associated with parabolas. This includes understanding the standard form of a quadratic equation and how it relates to the graph of a parabola. Once familiar with these concepts, students can begin working through the worksheet, answering questions that may involve graphing parabolas, calculating specific points, or analyzing given equations. It is beneficial to work through the problems step by step, ensuring a clear understanding of each characteristic before moving on to the next.
Key elements of the 1 Worksheet Characteristics Of Parabolas
Key elements of the 1 Worksheet Characteristics Of Parabolas include:
- Vertex: The highest or lowest point of the parabola, depending on its orientation.
- Axis of symmetry: A vertical line that divides the parabola into two mirror-image halves.
- Direction of opening: Indicates whether the parabola opens upwards or downwards.
- Focus and directrix: Points that define the parabola's shape and position.
- Intercepts: Points where the parabola intersects the x-axis and y-axis.
Steps to complete the 1 Worksheet Characteristics Of Parabolas
Completing the 1 Worksheet Characteristics Of Parabolas involves several steps:
- Read through the instructions carefully to understand the requirements of each question.
- Identify the equation of the parabola provided in the worksheet.
- Determine the vertex by using the formula derived from the standard form of the equation.
- Calculate the axis of symmetry and the direction of the parabola's opening.
- Locate the focus and directrix, if applicable, using the properties of parabolas.
- Graph the parabola, marking key points such as the vertex and intercepts.
- Review your answers to ensure accuracy and completeness.
Examples of using the 1 Worksheet Characteristics Of Parabolas
Examples of using the 1 Worksheet Characteristics Of Parabolas can include:
- Graphing the parabola defined by the equation y = x² - 4, identifying its vertex at (0, -4).
- Finding the axis of symmetry for the equation y = -2x² + 3, which is x = 0.
- Calculating the focus and directrix for a parabola given in vertex form, such as y = (x - 2)² + 1.
Legal use of the 1 Worksheet Characteristics Of Parabolas
The 1 Worksheet Characteristics Of Parabolas is intended for educational purposes and can be legally used in classrooms, tutoring sessions, or for self-study. It is important to ensure that the worksheet is used in accordance with copyright laws and educational guidelines. Teachers and students should verify that any resources used are properly attributed and that any modifications made for educational use comply with relevant regulations.
Create this form in 5 minutes or less
Related searches to 1 Worksheet Characteristics Of Parabolas
Create this form in 5 minutes!
How to create an eSignature for the 1 worksheet characteristics of parabolas
How to create an electronic signature for a PDF online
How to create an electronic signature for a PDF in Google Chrome
How to create an e-signature for signing PDFs in Gmail
How to create an e-signature right from your smartphone
How to create an e-signature for a PDF on iOS
How to create an e-signature for a PDF on Android
People also ask
-
What is the '1 Worksheet Characteristics Of Parabolas' feature in airSlate SignNow?
The '1 Worksheet Characteristics Of Parabolas' feature in airSlate SignNow allows users to create and manage worksheets that focus on the essential properties of parabolas. This feature is designed to enhance learning and understanding of parabolic characteristics, making it an excellent tool for educators and students alike.
-
How can I access the '1 Worksheet Characteristics Of Parabolas' in airSlate SignNow?
To access the '1 Worksheet Characteristics Of Parabolas,' simply sign up for an airSlate SignNow account. Once logged in, you can navigate to the templates section where you will find various educational resources, including the worksheet on parabolas.
-
Is there a cost associated with using the '1 Worksheet Characteristics Of Parabolas' feature?
airSlate SignNow offers a variety of pricing plans, including a free trial that allows you to explore features like the '1 Worksheet Characteristics Of Parabolas.' After the trial, you can choose a plan that fits your needs and budget, ensuring you get the best value for your educational resources.
-
What are the benefits of using airSlate SignNow for the '1 Worksheet Characteristics Of Parabolas'?
Using airSlate SignNow for the '1 Worksheet Characteristics Of Parabolas' provides several benefits, including easy document sharing, electronic signatures, and a user-friendly interface. These features streamline the process of distributing and collecting worksheets, making it easier for educators to manage their teaching materials.
-
Can I integrate airSlate SignNow with other educational tools for the '1 Worksheet Characteristics Of Parabolas'?
Yes, airSlate SignNow offers integrations with various educational tools and platforms, allowing you to enhance the functionality of the '1 Worksheet Characteristics Of Parabolas.' This means you can seamlessly connect with tools you already use, improving your workflow and efficiency.
-
How does airSlate SignNow ensure the security of my '1 Worksheet Characteristics Of Parabolas' documents?
airSlate SignNow prioritizes the security of your documents, including the '1 Worksheet Characteristics Of Parabolas.' With advanced encryption and secure cloud storage, you can trust that your educational materials are protected and accessible only to authorized users.
-
What types of users benefit from the '1 Worksheet Characteristics Of Parabolas' feature?
The '1 Worksheet Characteristics Of Parabolas' feature is beneficial for a wide range of users, including teachers, students, and educational administrators. It provides a structured approach to learning about parabolas, making it an essential resource for anyone involved in mathematics education.
Get more for 1 Worksheet Characteristics Of Parabolas
- Babysitters list form
- Board resolution format for companies to be indusind bank
- Active reading section 2 the ozone shield form
- Georgia realtors rental application form
- Fbla impromptu speaking topics form
- Brazoria county indigent program form
- College of education ctu online ctu online edu form
- Polyoak online application form
Find out other 1 Worksheet Characteristics Of Parabolas
- Electronic signature Alabama Courts Credit Memo Mobile
- Electronic signature Alabama Courts Credit Memo Now
- Electronic signature Alabama Courts Credit Memo Later
- Electronic signature Missouri Sports Letter Of Intent Later
- Electronic signature Alabama Courts Credit Memo Myself
- Electronic signature Alabama Courts Credit Memo Free
- Electronic signature Alabama Courts Credit Memo Secure
- Electronic signature Missouri Sports Letter Of Intent Myself
- Electronic signature Alabama Courts Credit Memo Fast
- How To Electronic signature Alabama Courts Credit Memo
- Electronic signature Alabama Courts Credit Memo Simple
- Electronic signature Alabama Courts Credit Memo Easy
- Electronic signature Alabama Courts Credit Memo Safe
- Electronic signature Missouri Sports Letter Of Intent Free
- How Do I Electronic signature Alabama Courts Credit Memo
- Help Me With Electronic signature Alabama Courts Credit Memo
- Electronic signature Missouri Sports Letter Of Intent Secure
- Electronic signature Missouri Sports Rental Application Online
- How Can I Electronic signature Alabama Courts Credit Memo
- Electronic signature Missouri Sports Rental Application Computer