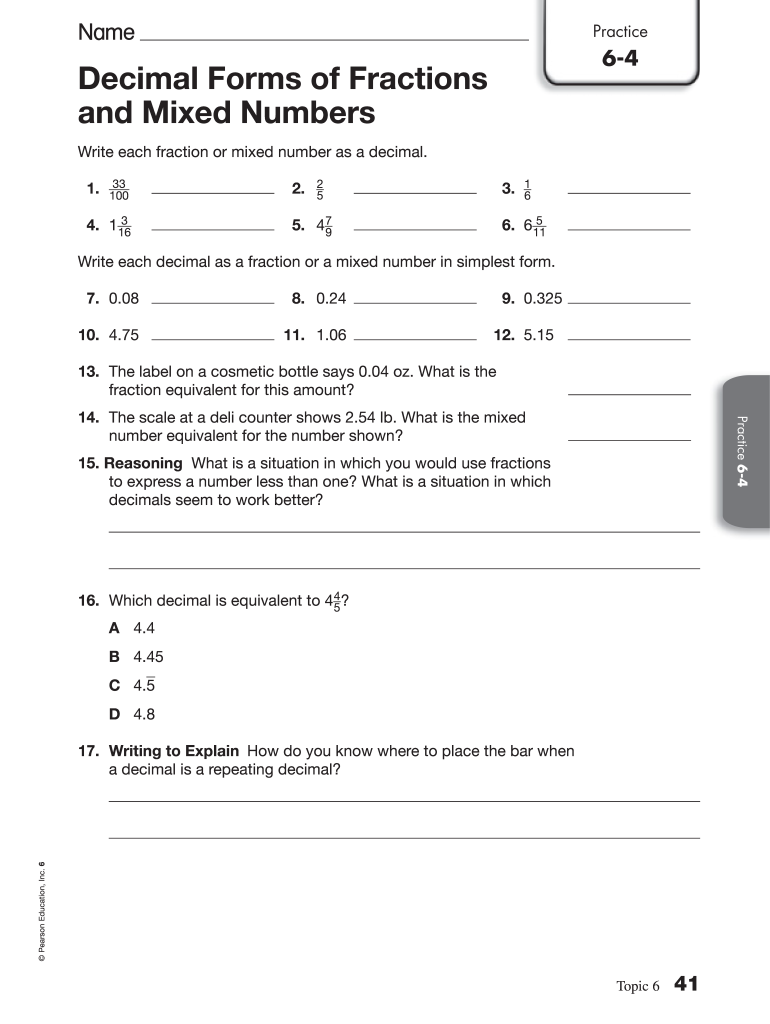
Decimal Forms of Fractions and Mixed Numbers Practice 6 4

Convert to common denominators using cross-multiplication.Answer, from smallest to largest: 12/25, 24/49, 36/73.To compare a/b and c/d, convert to (a*d)/(b*d) and (c*b)/(d*b).(The common denominator is found by multiplying the denominators [bottom numbers] together “b*d”, and to keep the ratios consistent, we also multiple the numerators [top numbers] by the the “other” denominators [b or d], as “a*d” and “c*b”)For example 2/3 and 4/5 converts to (2*5)/(3*5) and (4*3)/(5*3) or 10/15 and 12/15, since the denominators are the same (15) and numerator 12 > 10, we know that 4/5 (“12/15”) is greater than 2/3 (“10/15”).In the example we can expand the formula to a/b, c/d, e/f to (a*d*f)/(b*d*f), (c*b*f)/(b*d*f), (e*b*d)/(b*d*f) or (24*73*25)/(49*73*25), (36*49*25)/(49*73*25), (12*49*73)(23*73*25) == 43800/89425, 44100/89425, 42924/89425

None of the above?

There should be a s<->d button not far above AC. Shift + that button gets you a mixed fraction, just that button gets you between fractions and decimals. If you want it to display decimals automatically the first time just reset the calculator.Hope this helps :)

There are two ways to solve it:-(5C3)*(3!) = 60.3 digits out of 5 can be chosen in (5C3) ways and (3!) being the permutation of the three digits chosen in a given choice among themselves.2. 5*4*3 = 60.Since, the number to be formed will be having 3 digits. So, the hundred’s place can be filled in 5 ways, ten’s place in 4 ways (for every formation of the hundred’s digit) and one’s place in 3 ways (for every formation of the hundred’s and ten’s digits).

Too lazy for homework?if a number is divisible by 5, how it should end?by 1,2,3,3,4,5 or 6?thus the last number is fixed.First can be any of 5.Second can be any of remaining 4.Third can be any of remaining 3.Forth can be any of remaining 2.Thus one has 120 possibilities.

So every decimal from 0–0.25 and to one decimal place let's look at all decimal places that the number could round to: 0, 0.1, 0.2, 0.3 okay let's star with zero 0.000~1 to 0.0444444~ will round down so 1/2 or 50%to give you a good answer and the same 50% rule will follow through out so your final answer is 1/2 of all possible numbers

You are asking for the domination number[1] of a certain Johnson graph[2] (please, no Big Lebowski jokes). In standard notation, this number is denoted [math]\gamma(J(90,5))[/math].(Note: I'm assuming that the “5 numbers drawn out of 90” are all different, which I believe is the case in most lotteries).The Johnson graph [math]J(90,5)[/math] is the graph whose vertices correspond to all the [math]5[/math]-element subsets of [math]\{1,2,\ldots,90\}[/math], and two vertices are adjacent (connected with an edge) whenever the corresponding sets have [math]4[/math] elements in common. For example, [math]\{2,3,5,8,13\}[/math] is adjacent to [math]\{2,3,8,13,77\}[/math] but not to [math]\{2,3,5,9,14\}[/math].A dominating set for a graph is a set of vertices that is adjacent to each vertex not in the set. If you have a dominating set of tickets for [math]J(90,5)[/math], whatever the winning combination turns out to be has at least [math]4[/math] matches with a ticket in your hand.Calculating the size of a minimal dominating set, called the domination number, is computationally hard (the corresponding decision problem is NP-complete), and our graph [math]G=J(90,5)[/math] is no peanut: it has [math]N=\binom{90}{5}=43\,949\,268[/math] vertices, each with [math]d=5(90-5)=425[/math] neighbors.Of course, [math]G[/math] is a very specific and highly symmetric graph, so it's entirely possible that a minimal dominating set for it, or at least a relatively small dominating set, can be determined explicitly using some reasoning particular to this case.As with any optimization problem, we can pursue two competing paths: we may seek upper bounds by exhibiting some good dominating sets, and we can seek lower bounds by attempting to prove that every dominating set must be at least as large as some size [math]B[/math].Since the degree of [math]G[/math] is [math]425[/math], a dominating set must have at least [math]N/425[/math] elements, which sets a lower bound of [math]B=103\,411[/math] tickets. With fewer tickets than this you stand no chance of guaranteeing a four-element match. I suspect there is, in fact, a dominating set of this size, or very close to it.An equally obvious upper bound is obtained by simply scanning all [math]4[/math]-number combinations [math](a,b,c,d)[/math] in our range and filling in the fifth number arbitrarily. This yields a dominating set of size [math]\binom{90}{4}[/math]. That's about 2.5 million tickets, which is far more than the theoretical lower bound, but it's also far less than the 43 million possible winning combinations.Of course, this is very wasteful. We made no use of the fact that each ticket can cover five [math]4[/math]-number combinations, not just one. A better approach is to use a standard trick in design theory or coding theory: impose a linear constraint.Specifically, consider those combinations where the sum of the numbers is divisible by [math]90[/math]. About [math]1/90[/math] of the combinations are special like that (The exact number is [math]488\,326[/math], which is just [math]N/90[/math] rounded up).Suppose you filled tickets with all of these special combinations. If the winning one happens to be special, you got it. If it's not, then in almost all cases it has four numbers in common with not only one but five of your special combinations.The reason is that given any winning combination [math]\{a,b,c,d,e\}[/math], you can keep [math]a,b,c[/math] and [math]d[/math] intact and find an appropriate fifth number [math]x[/math] (instead of [math]e[/math]) which makes the sum [math]a+b+c+d+x[/math] divisible by [math]90[/math]. The combination [math]\{a,b,c,d,x\}[/math] is then in your hand, unless it so happens that [math]x[/math] is identical to one of [math]a,b,c,d[/math]. In this case you can try picking another element of the winning combo, say [math]d[/math], and try [math]\{a,b,c,y,e\}[/math] whose sum is divisible by [math]90[/math]. Once again, we just need to hope that [math]y[/math] isn't identical to one of the other four numbers.For example, suppose the winning combination was [math]\{1,2,3,42,60\}[/math]. The quadruplet [math]\{1,2,3,42\}[/math] is nowhere to be found among the special combinations, because the missing fifth number would have been [math]42[/math] itself. However, that's ok because [math]\{1,2,3,24,60\}[/math] is special, and matches four of the numbers in the winning combination. We can't “fix” the [math]60[/math], but we can fix any of the other numbers.In very rare instances, a winning combination may not be “fixable” at all. For example, suppose that the lottery was played with three numbers instead of five. The winning combination [math]\{10,40,70\}[/math] cannot be modified into a special combination by changing any single number.If that had happened in our case, we would simply have added a few tickets to cover for those pathological cases. But I'm quite sure that's not necessary: as far as I can tell there aren’t any “bad” combinations of five numbers in the range 1 through 90.The set of special tickets guarantees winning the lottery, but it’s still about 4.7x larger than the theoretical lower bound. This isn't surprising because, as we said, most winning combinations are actually covered by 5 of our tickets, though sometimes it's just 4. That's where this factor of 4.7 comes from.We can seek various pruning techniques to find winning sets of fewer tickets, but I couldn't yet find one that'll take you down to around 110,000 tickets. I'm 100% sure this is addressed in the literature, and I'll update this answer if I find anything.Footnotes[1] Dominating set - Wikipedia[2] Johnson graph - Wikipedia

I’m going to assume that different fractions that have the same value (like 1/3 and 2/6) are allowed. So, the denominator can be any of 5 numbers, and the numerator any of 4 numbers. So, I’d say there are 5*4 possible fractions that can be formed (or 20). (That is assuming that both numerator and denominator are single digits —- 1/2, 1/3, 1/6, 1/8, 1/9, 2/2, 2/3, 2/6, 2/8, 2/9 ——- etc.)

airSlate SignNow provides a range of features to streamline the signing process, including templates, document editing, and team collaboration tools. These features are essential in understanding which fraction and decimal forms match the long division problem, making it easier to integrate educational materials into your workflow.

The platform employs advanced encryption and compliant storage methods to ensure document safety. By understanding which fraction and decimal forms match the long division problem, users can confidently share sensitive educational content without compromising security.

Yes, airSlate SignNow offers a free trial for new users to explore its features without commitment. This is an excellent opportunity for educators and businesses to see how the platform can help them identify which fraction and decimal forms match the long division problem.

airSlate SignNow provides flexible pricing plans suitable for various business needs, starting from individual to enterprise solutions. Understanding which fraction and decimal forms match the long division problem will aid in maximizing the value of these plans for educational purposes.

Yes, airSlate SignNow seamlessly integrates with a variety of applications including Google Workspace and Slack. This allows users to easily access and analyze data related to which fraction and decimal forms match the long division problem within their favorite tools.

airSlate SignNow enhances efficiency by simplifying the document signing process, which saves time and reduces costs. Understanding which fraction and decimal forms match the long division problem can further improve educational outsignNow and efficiency for businesses focused on learning.

Absolutely! airSlate SignNow is optimized for mobile use, allowing users to sign and send documents on the go. This flexibility helps educators teach concepts like which fraction and decimal forms match the long division problem from anywhere.